- 1.6 Limit Based Continuityap Calculus 2nd Edition
- 1.6 Limit Based Continuityap Calculus Algebra
- 1.6 Limit Based Continuityap Calculus Calculator
SAT Math Test Prep Online Crash Course Algebra & Geometry Study Guide Review, Functions,Youtube - Duration: 2:28:48. The Organic Chemistry Tutor 1,203,506 views. Calculus 1 - Supplemental Chapter 1.6 Material. An appropriate substitution to convert the limit problem into one which is easier to solve based on known facts. Unit: Limits and continuity. Unit: Limits and continuity. Legend (Opens a modal). Limits at infinity of quotients with square roots (odd. If you have a ‘bad' limit, always look for some cancellation of factors in the numerator and denominator. In fact, for hundreds of years people only evaluated limits in this style! After all, human beings can't really execute infinite limiting processes, and so on.
Quick Overview
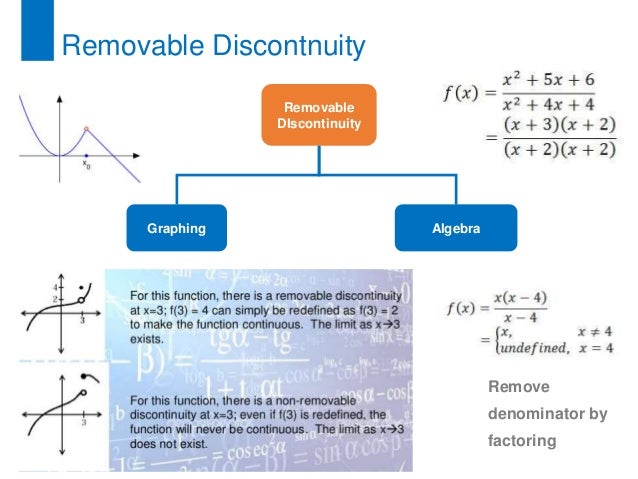
- Discontinuities can be classified as jump, infinite, removable, endpoint, or mixed.
- Removable discontinuities are characterized by the fact that the limit exists.
- Removable discontinuities can be 'fixed' by re-defining the function.
- The other types of discontinuities are characterized by the fact that the limit does not exist. Specifically,
- Jump Discontinuities: both one-sided limits exist, but have different values.
- Infinite Discontinuities: both one-sided limits are infinite.
- Endpoint Discontinuities: only one of the one-sided limits exists.
- Mixed: at least one of the one-sided limits does not exist.
Jump Discontinuities



Game 60: july 31 2015 the initials game 6. The graph of $$f(x)$$ below shows a function that is discontinuous at $$x = a$$.
1.6 Limit Based Continuityap Calculus 2nd Edition
In this graph, you can easily see that $$ {color{importantColor}limlimits_{xto a^-} f(x) = L} % mbox{ and } % {color{secondaryColor}limlimits_{xto a^+} f(x) = M}. $$
The function is approaching different values depending on the direction $$x$$ is coming from. When this happens, we say the function has a jump discontinuity at $$x=a$$.
1.6 Limit Based Continuityap Calculus Algebra
Infinite Discontinuities
The graph below shows a function that is discontinuous at $$x=a$$.
The arrows on the function indicate it will grow infinitely large as $$x$$ approaches $$a$$. Since the function doesn't approach a particular finite value, the limit does not exist. This is an infinite discontinuity.
The following two graphs are also examples of infinite discontinuities at $$x = a$$. Notice that in all three cases, both of the one-sided limits are infinite.
Removable Discontinuities
In the graphs below, there is a hole in the function at $$x=a$$. These holes are called removable discontinuities
Notice that for both graphs, even though there are holes at $$x = a$$, the limit value at $$x=a$$ exists.
Removable Discontinuities can be Fixed
Removable discontinuities can be fixed by redefining the function, as shown in the following example.
Example
The function below has a removable discontinuity at $$x = 2$$. Redefine the function so that it becomes continuous at $$x=2$$.
$$f(x) = frac{x^2-2x}{x^2-4}$$
SolutionThe graph of the function is shown below for reference.
In order to fix the discontinuity, we need to know the $$y$$-value of the hole in the graph. To determine this, we find the value of $$limlimits_{xto 2} f(x)$$.
Examining the form of the limit we see
$$displaystylelim_{xto 2} frac{x^2-2x}{x^2-4} = frac{(2)^2 - 2(2)}{(2)^2-4} = frac 0 0$$
The division by zero in the $$frac 0 0$$ form tells us there is definitely a discontinuity at this point.
Next, using the techniques covered in previous lessons (see Indeterminate Limits---Factorable) we can easily determine
$$displaystylelim_{xto 2} f(x) = frac 1 2$$
The limit value is also the $$y$$-value of the hole in the graph. Now we can redefine the original function in a piecewise form:
$$ f(x) = left{% begin{array}{ll} frac{x^2-2x}{x^2-4}, & mbox{for all } x neq 2[6pt] % frac 1 2, & mbox{for } x = 2 end{array} right. $$
The first piece preserves the overall behavior of the function, while the second piece plugs the hole.
Endpoint Discontinuities
When a function is defined on an interval with a closed endpoint, the limit cannot exist at that endpoint. This is because the limit has to examine the function values as $$x$$ approaches from both sides.
For example, consider finding $$displaystylelimlimits_{xto0} sqrt x$$ (see the graph below).

- Discontinuities can be classified as jump, infinite, removable, endpoint, or mixed.
- Removable discontinuities are characterized by the fact that the limit exists.
- Removable discontinuities can be 'fixed' by re-defining the function.
- The other types of discontinuities are characterized by the fact that the limit does not exist. Specifically,
- Jump Discontinuities: both one-sided limits exist, but have different values.
- Infinite Discontinuities: both one-sided limits are infinite.
- Endpoint Discontinuities: only one of the one-sided limits exists.
- Mixed: at least one of the one-sided limits does not exist.
Jump Discontinuities
Game 60: july 31 2015 the initials game 6. The graph of $$f(x)$$ below shows a function that is discontinuous at $$x = a$$.
1.6 Limit Based Continuityap Calculus 2nd Edition
In this graph, you can easily see that $$ {color{importantColor}limlimits_{xto a^-} f(x) = L} % mbox{ and } % {color{secondaryColor}limlimits_{xto a^+} f(x) = M}. $$
The function is approaching different values depending on the direction $$x$$ is coming from. When this happens, we say the function has a jump discontinuity at $$x=a$$.
1.6 Limit Based Continuityap Calculus Algebra
Infinite Discontinuities
The graph below shows a function that is discontinuous at $$x=a$$.
The arrows on the function indicate it will grow infinitely large as $$x$$ approaches $$a$$. Since the function doesn't approach a particular finite value, the limit does not exist. This is an infinite discontinuity.
The following two graphs are also examples of infinite discontinuities at $$x = a$$. Notice that in all three cases, both of the one-sided limits are infinite.
Removable Discontinuities
In the graphs below, there is a hole in the function at $$x=a$$. These holes are called removable discontinuities
Notice that for both graphs, even though there are holes at $$x = a$$, the limit value at $$x=a$$ exists.
Removable Discontinuities can be Fixed
Removable discontinuities can be fixed by redefining the function, as shown in the following example.
Example
The function below has a removable discontinuity at $$x = 2$$. Redefine the function so that it becomes continuous at $$x=2$$.
$$f(x) = frac{x^2-2x}{x^2-4}$$
SolutionThe graph of the function is shown below for reference.
In order to fix the discontinuity, we need to know the $$y$$-value of the hole in the graph. To determine this, we find the value of $$limlimits_{xto 2} f(x)$$.
Examining the form of the limit we see
$$displaystylelim_{xto 2} frac{x^2-2x}{x^2-4} = frac{(2)^2 - 2(2)}{(2)^2-4} = frac 0 0$$
The division by zero in the $$frac 0 0$$ form tells us there is definitely a discontinuity at this point.
Next, using the techniques covered in previous lessons (see Indeterminate Limits---Factorable) we can easily determine
$$displaystylelim_{xto 2} f(x) = frac 1 2$$
The limit value is also the $$y$$-value of the hole in the graph. Now we can redefine the original function in a piecewise form:
$$ f(x) = left{% begin{array}{ll} frac{x^2-2x}{x^2-4}, & mbox{for all } x neq 2[6pt] % frac 1 2, & mbox{for } x = 2 end{array} right. $$
The first piece preserves the overall behavior of the function, while the second piece plugs the hole.
Endpoint Discontinuities
When a function is defined on an interval with a closed endpoint, the limit cannot exist at that endpoint. This is because the limit has to examine the function values as $$x$$ approaches from both sides.
For example, consider finding $$displaystylelimlimits_{xto0} sqrt x$$ (see the graph below).
Note that $$x=0$$ is the left-endpoint of the functions domain: $$[0,infty)$$, and the function is technically not continuous there because the limit doesn't exist (because $$x$$ can't approach from both sides).
We should note that the function is right-hand continuous at $$x=0$$ which is why we don't see any jumps, or holes at the endpoint.
Mixed Discontinuities
Consider the graph shown below. Insite 7,6 keygen.
The function is obviously discontinuous at $$x = 3$$. From the left, the function has an infinite discontinuity, but from the right, the discontinuity is removable. Since there is more than one reason why the discontinuity exists, we say this is a mixed discontinuity
1.6 Limit Based Continuityap Calculus Calculator
When you work with limit and continuity problems in calculus, there are a couple of formal definitions you need to know about. So, before you take on the following practice problems, you should first re-familiarize yourself with these definitions.
Here is the formal, three-part definition of a limit:
For a function f (x) and a real number a,
exists if and only if
(Note that this definition does not apply to limits as x approaches infinity or negative infinity.)
Now, here's the definition of continuity:
A function f (x) is continuous at a point a if three conditions are satisfied:
Now it's time for some practice problems.
Practice questions
Using the definitions and this figure, answer the following questions.
At which of the following x values are all three requirements for the existence of a limit satisfied, and what is the limit at those x values?
x = –2, 0, 2, 4, 5, 6, 8, 10, and 11.
At which of the x values are all three requirements for continuity satisfied?
Answers and explanations
All three requirements for the existence of a limit are satisfied at the x values 0, 4, 8, and 10:
At 0, the limit is 2.
At 4, the limit is 5.
At 8, the limit is 3.
At 10, the limit is 5.
To make a long story short, a limit exists at a particular x value of a curve when the curve is heading toward some particular y value and keeps heading toward that y value as you continue to zoom in on the curve at the x value. The curve must head toward that y value (that height) as you move along the curve both from the right and from the left (unless the limit is one where x approaches infinity).
The phrase heading toward is emphasized here because what happens precisely at the given x value isn't relevant to this limit inquiry. That's why there is a limit at a hole like the ones at x = 8 and x = 10.
The function in the figure is continuous at 0 and 4.
The common-sense way of thinking about continuity is that a curve is continuous wherever you can draw the curve without taking your pen off the paper. It should be obvious that that's true at 0 and 4, but not at any of the other listed x values.
